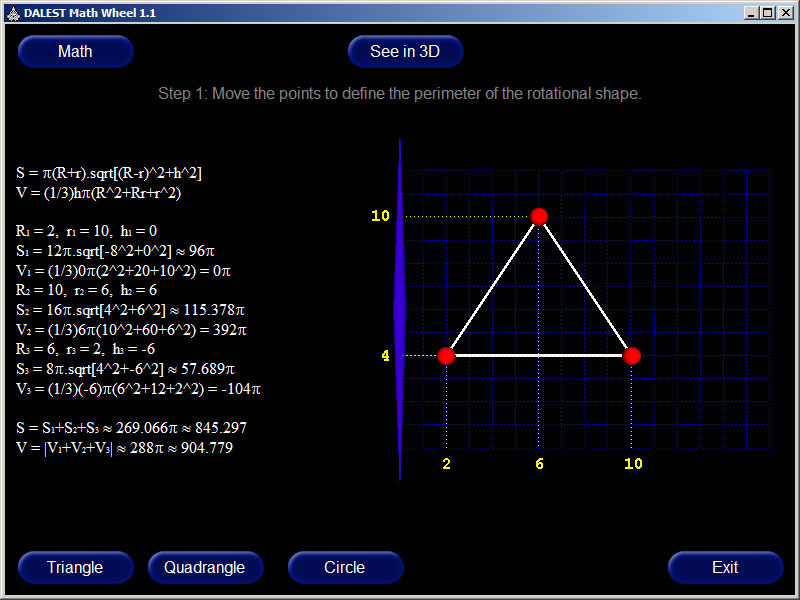
The Math Wheel is aDALESTapplication. It is similar to thePotter's Wheelapplication. The user has three main objects – a triangle, a quadrangle and a circle. All their vertices can be freely dragged and the volume and surface of the rotational figures can be calculated along with displaying of the "algorithm"

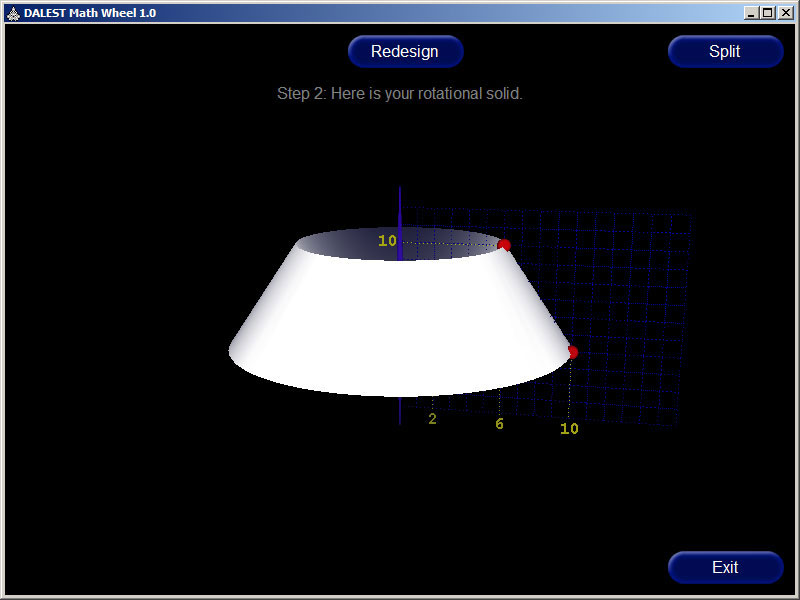
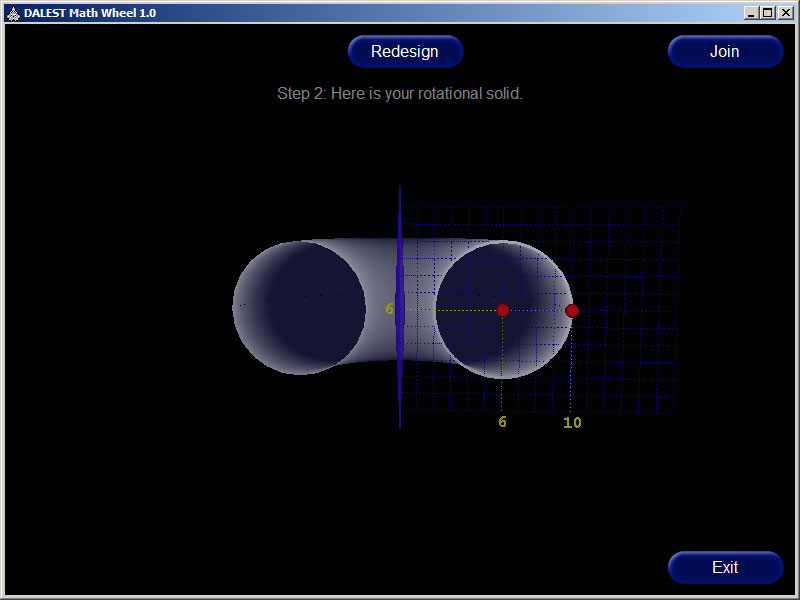
1. Study the volume and area of circle-based rotational objects. How does they depend on the size and the position of the circle?
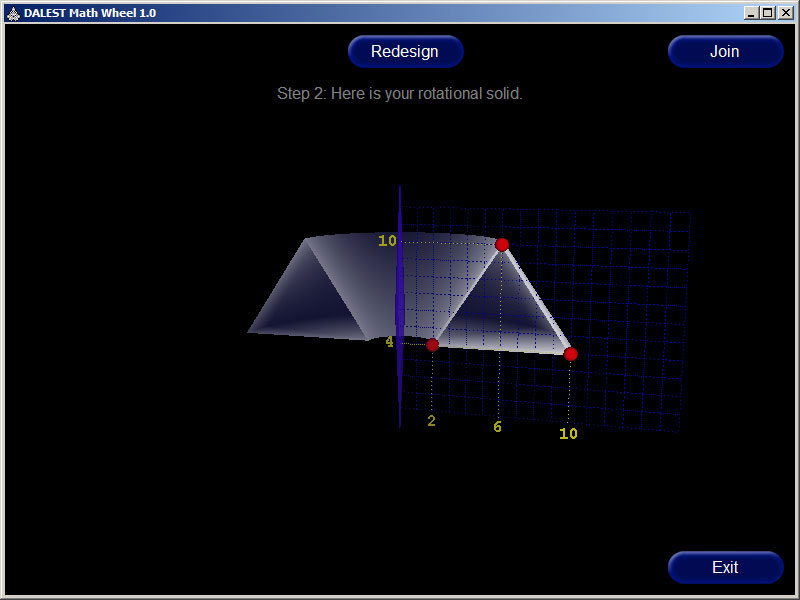
2. Study the volume and area of triangle-based rotational objects; experiment with various types of triangles and orientations
3. Study the volume and area of quadrangle-based rotational objects; experiment with various types of triangles and orientations
4. Analyze the mathematical symbolic explanation of volume and area; try the find the reasoning or the method behind the formulae
5. Choose a number for a volume and/or area, then build a rotational object with such volume and area; do this experimentally (by trial and error) and then analytically (by calculating the position)
6. Find alternative methods for calculating the volume and area. Are these methods applicable to figures with more than 4 vertices?
7. Produce simpler formulae for some specific cases like non-rotated square, right-triangle, and so on
8. Use the Math Wheel to find the formulae for volume and area of a cylinder, a cone, a truncated cone
9. Using the circle as a base figure, find a situation when the number for the volume of the torus is the same as the number of the area of the torus
10. Find pairs of rotational objects with the same volume (or area) but based on different 2D figures
11. Find rotational objects around you (buildings, vases, bottles, etc) and try to calculate their volume and surface
12. Design rotational objects with zero volume and zero area, but with distinct vertices (i.e. no two vertices share the same position)
13. Design rotational objects with zero volume and non-zero area, but with distinct vertices
14. Calculate the volume and area of a circle (hint: circle is not a disk and is not a torus)
15. Explain the role of |.| in the formulae of volume of objects based on polygons. Give examples when the absolute value is not needed, and counter examples when it is needed. What does affect the sign of the volume?
16. Invent the formulae for the volume of a pie cut out from a rotational object
17. Invent the formulae for the area of a pie cut out from a rotational object including/excluding the area of the intersection
18. Try to derive formulae for volume and area of rotational objects using the area and perimeter of their 2D figures. What other parameters are needed?